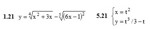IDZ Ryabushko Option 21 KR "Derivatives and their appli
Content: 21v-KR_proizvodniye.pdf (111.41 KB)
Uploaded: 20.02.2025
Positive responses: 0
Negative responses: 0
Sold: 0
Refunds: 0
$1.47
Test "Derivatives and their applications" (2 hours). 260 pages
1. Find the first derivative of y′
2. Find the first derivative of y′
2.21 y = (1 + ex)/(1 – ex)
3. Calculate the first derivative of the function for the specified value of the argument or parameter or for the given coordinates of the point.
3.21 y(x) = (1 + x3)(5 – 1/ x2), x = 1, x = 0
4. Find the second derivative of y′′
4.21 y = arctan(2x/(1 – x2))
5. Find the second derivative d2y/dx2 of the function.
6. Solve the following problems.
6.21. Write the equation of the normal to the astroid x = acos3t, y = asin3t at the point for which t = π/4.
7. Solve the following problems.
7.21. The radius of a sphere increases uniformly at a rate of 5 cm/s. At what rate do the surface area of the sphere and its volume increase at the moment when its radius becomes equal to 50 cm?
1. Find the first derivative of y′
2. Find the first derivative of y′
2.21 y = (1 + ex)/(1 – ex)
3. Calculate the first derivative of the function for the specified value of the argument or parameter or for the given coordinates of the point.
3.21 y(x) = (1 + x3)(5 – 1/ x2), x = 1, x = 0
4. Find the second derivative of y′′
4.21 y = arctan(2x/(1 – x2))
5. Find the second derivative d2y/dx2 of the function.
6. Solve the following problems.
6.21. Write the equation of the normal to the astroid x = acos3t, y = asin3t at the point for which t = π/4.
7. Solve the following problems.
7.21. The radius of a sphere increases uniformly at a rate of 5 cm/s. At what rate do the surface area of the sphere and its volume increase at the moment when its radius becomes equal to 50 cm?
Detailed solution. Designed in PDF format for easy viewing of IDZ solutions on smartphones. In MS Word (doc format) sent additionally.
No feedback yet