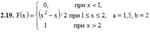DHS 18.2 - Option 19. Decisions Ryabushko AP
Content: 19v-IDZ18.2.pdf (160.13 KB)
Uploaded: 10.07.2025
Positive responses: 0
Negative responses: 0
Sold: 8
Refunds: 0
$1.47
1. Find the distribution of the said discrete CB X and its distribution function F (x). Calculate the expectation M (X), the variance of D (X) and standard deviation σ (X). Draw the graph of the distribution function F (x)
1.19. Work serves four machine. The probability of failure for a change for the first machine is 0.6, for the second - 0.5, for the third - 0.4 for the fourth - 0.5; SW X - the number of machines that fail per shift.
2. Dana distribution function F (x) DM X. Find the probability density function f (x), the expectation M (X), the variance of D (X), and the probability of hitting NE X on the interval [a; b]. Construct the graphs of the functions F (x) and f (x).
3. Solve the following problems.
3.19. At a predetermined position of the projectile point of rupture goal is covered with a Poisson field with debris density λ = 2,5 fragments / m2. Projection target area on the plane on which the observed fragmentation field is 0.8 m2. Each shard hit the target, striking it with complete certainty. Find the probability that the target will be destroyed.
4. Solve the following problems.
4.19. The variance of each of the 2,500 independent CB does not exceed 5. Assess the probability that the deviation of the arithmetic mean of these random values from their arithmetic mean of the expectations will not exceed 0.4.
1.19. Work serves four machine. The probability of failure for a change for the first machine is 0.6, for the second - 0.5, for the third - 0.4 for the fourth - 0.5; SW X - the number of machines that fail per shift.
2. Dana distribution function F (x) DM X. Find the probability density function f (x), the expectation M (X), the variance of D (X), and the probability of hitting NE X on the interval [a; b]. Construct the graphs of the functions F (x) and f (x).
3. Solve the following problems.
3.19. At a predetermined position of the projectile point of rupture goal is covered with a Poisson field with debris density λ = 2,5 fragments / m2. Projection target area on the plane on which the observed fragmentation field is 0.8 m2. Each shard hit the target, striking it with complete certainty. Find the probability that the target will be destroyed.
4. Solve the following problems.
4.19. The variance of each of the 2,500 independent CB does not exceed 5. Assess the probability that the deviation of the arithmetic mean of these random values from their arithmetic mean of the expectations will not exceed 0.4.
Detailed solution. Designed in PDF format for easy viewing of IDZ solutions on smartphones and PCs. In MS Word (doc format) sent additionally.
No feedback yet