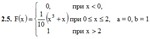DHS 18.2 - Option 5. Decisions Ryabushko AP
Content: 5v-IDZ18.2.pdf (151.40 KB)
Uploaded: 10.07.2025
Positive responses: 0
Negative responses: 0
Sold: 9
Refunds: 0
$1.47
1. Find the distribution of the said discrete CB X and its distribution function F (x). Calculate the expectation M (X), the variance of D (X) and standard deviation σ (X). Draw the graph of the distribution function F (x)
1.5. The probability of the release of the device, satisfying the requirements of quality, equal to 0.9. In the inspection lot - 3 device; SW X - the number of devices that meet the quality requirements.
2. Dana distribution function F (x) DM X. Find the probability density function f (x), the expectation M (X), the variance of D (X), and the probability of hitting NE X on the interval [a; b]. Construct the graphs of the functions F (x) and f (x).
3. Solve the following problems.
3.5. Graduation meter scale is 0.2. Instrument readings are rounded to the nearest whole division. Assuming that the measurement errors are distributed evenly, find the probability that the error count, the smaller will be made 0.04.
4. Solve the following problems.
4.5. SW is the average of independent and identically distributed random variables, the variance of each of which is equal to 5. How long does it take such quantities to DM X with probability not less than 0.9973, deviated from its mathematical expectation is not more than 0.01?
1.5. The probability of the release of the device, satisfying the requirements of quality, equal to 0.9. In the inspection lot - 3 device; SW X - the number of devices that meet the quality requirements.
2. Dana distribution function F (x) DM X. Find the probability density function f (x), the expectation M (X), the variance of D (X), and the probability of hitting NE X on the interval [a; b]. Construct the graphs of the functions F (x) and f (x).
3. Solve the following problems.
3.5. Graduation meter scale is 0.2. Instrument readings are rounded to the nearest whole division. Assuming that the measurement errors are distributed evenly, find the probability that the error count, the smaller will be made 0.04.
4. Solve the following problems.
4.5. SW is the average of independent and identically distributed random variables, the variance of each of which is equal to 5. How long does it take such quantities to DM X with probability not less than 0.9973, deviated from its mathematical expectation is not more than 0.01?
Detailed solution. Designed in PDF format for easy viewing of IDZ solutions on smartphones and PCs. In MS Word (doc format) sent additionally.
No feedback yet